Chapter 4: Water Vapor
Alison Nugent and Shintaro Russell
Learning Objectives
By the end of this chapter, you should be able to:
- Compute saturation vapor pressure using the Clausius-Clapeyron equation;
- Convert between humidity variables. Differentiate between relative humidity, specific humidity, absolute humidity, wet-bulb temperature, mixing ratio, and dew point;
- Describe the conditions for saturation to occur;
- Apply the moist adiabatic lapse rate;
- Use the principles of phase change and latent heating to describe why the moist adiabatic lapse rate is less than the dry adiabatic lapse rate.
Introduction
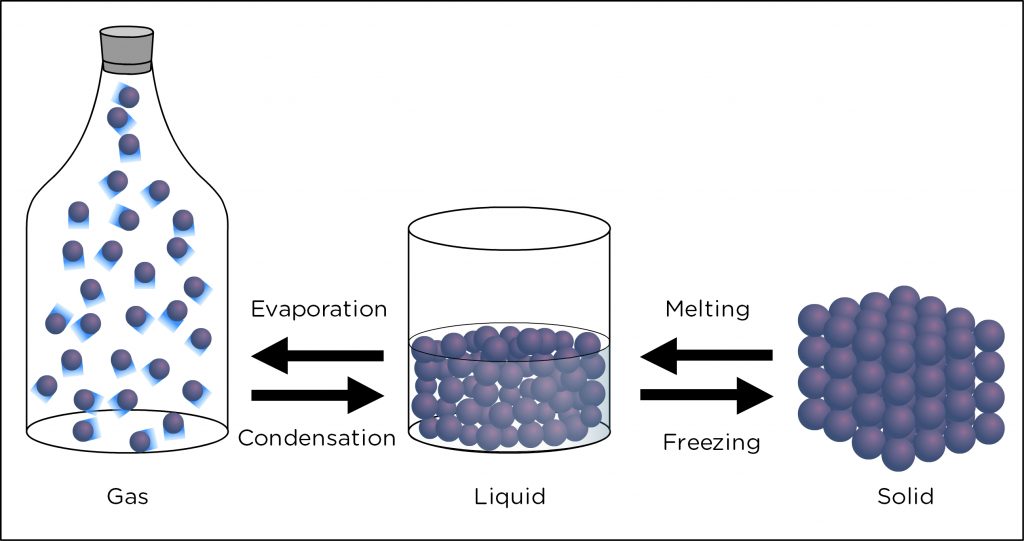
Water can exist as a solid, liquid, or gas at typical conditions found on Earth. As we learned, the process of liquid water becoming water vapor is called evaporation and this process absorbs or requires energy. The opposite process is called condensation, where water vapor becomes liquid water, releasing energy. Condensation is especially important in atmospheric science because this is the process that allows clouds to form.
Clouds are composed of millions and billions of tiny liquid water droplets. How do they form? Why are they there?

Before we can understand clouds in the atmosphere, we need to explore concepts like how humidity is defined and what saturation means.
In general, humidity is the amount of water vapor in the air. You’ve likely heard of relative humidity and dew point temperature, but what do these quantities mean physically?
Saturation
Imagine a closed jar filled halfway with water. At the initial time, more water molecules evaporate from the water surface than the number that return. However, after some time, the number of molecules evaporating from the surface will be equal to the number of molecules condensing back into the water surface. When condensation and evaporation are equal, this is called saturation.
Saturation occurs when air contains the maximum amount of water vapor possible for its given temperature. That is why condensation equals evaporation. If evaporation occurs, the air cannot contain more water vapor, so some must condense. Now let’s get quantitative.
Vapor pressure at saturation
Every gas in the atmosphere exerts pressure, for example, vapor pressure makes up a fraction of the total atmospheric pressure. In the following equation, all of the gases in Earth’s atmosphere contribute to the total atmospheric pressure Patmosphere.
![]()
Specifically for water vapor, the more water vapor that is added to the atmosphere, the higher the vapor pressure PH2O. The units for vapor pressure are the same as pressure and can be in Pascals, hectoPascals, or kiloPascals. Because we are staying consistent with Roland Stull’s Practical Meteorology textbook, we will use kiloPascals (kPa) throughout this chapter.
The amount of water vapor that the atmosphere can contain depends on temperature. Lower temperature air cannot contain as much water vapor as higher temperature air. If we think of this quantitatively in terms of pressure, saturation vapor pressure refers to the pressure exerted by the movement of water vapor molecules exerted over a surface of liquid water. When the partial pressure exerted by water vapor is equal to the saturation vapor pressure, the air is saturated.
The Clausius-Clapeyron equation gives the approximate relationship between saturation vapor pressure (es) and temperature in the atmosphere
![]()
where the water-vapor gas constant ℜv is 461 J·K–1·kg–1, T0 is 273.15 K, e0 is 0.6113 kPa, and Lv is the latent heat of vaporization, 2.5×106 J·kg–1. This results in Lv/ℜv being equal to 5423 K. In this equation, units for temperature must be in Kelvin. Note that in the equation above, exp[x] implies the exponential function ex, but it is written on one line for visual purposes.

The image shows the relationship between temperature and saturation vapor pressure based on the Clausius-Clapeyron equation. Lower temperatures are saturated with respect to water vapor at lower vapor pressures, while higher temperatures need higher vapor pressures to be saturated. Temperature is the primary factor determining water vapor saturation.
Humidity Variables
Vapor pressure is one way of defining humidity, but there are many others. Here is a non-comprehensive list of humidity variables and their typical units.
e = vapor pressure (kPa)
r = mixing ratio (g·kg–1)
q = specific humidity (g·kg–1)
ρv = absolute humidity (g·m-3)
RH = relative humidity (%)
zLCL = lifting condensation level (km)
Td = dew point (temperature) (°C)
Tw = wet-bulb temperature (°C)
Vapor Pressure
We’ve already discussed saturation vapor pressure, es, but you can also compute vapor pressure, e. However, because Td is often unknown, the easiest way is usually through relative humidity.
![]()
Again, e0 is 0.6113 kPa, Lv is 2.5×106 J·kg–1, ℜv is 461 J·K–1·kg–1, T0 is 273.15 K, and Td is dew point temperature, which will be defined later.
Mixing Ratio
Mixing ratio, r, is the ratio of the mass of water vapor to the mass of dry air. It is typically expressed as grams of water vapor per kilogram of air (g·kg–1).
![]()
![]()
Pressure (P) should be in the same units as vapor pressure (e). The constant ε is 0.622, is the ratio between the gas constant for dry air and the gas constant for water vapor.
![]()
Saturation mixing ratio, rs, is computed the same way as the mixing ratio but with saturation vapor pressure, es, instead of e.
![]()
When calculating mixing ratio, the pressure units on the top of the fraction will cancel with the pressure units on the bottom of the fraction. While it appears unit-less, its technically not based on its definition of mass of water vapor as compared to mass of dry air. See the Pro Tip below for more information.
Specific Humidity
Specific humidity, q, is the ratio of the mass of water vapor to the total mass of air (dry air and water vapor combined). It is expressed as grams of water vapor per kilogram of air (g·kg–1).
![]()
![]()
Again, saturation specific humidity, qs, is computed with es instead of e.
![]()
Absolute Humidity
Absolute humidity, ρv, is the ratio of the mass of water vapor to the volume of air. It is expressed as grams of water vapor in a cubic meter of air (g·m-3). It is effectively water vapor density.
![]()
![]()
Again, saturation absolute humidity, ρvs, uses es instead of e.
![]()
Relative Humidity
Relative humidity, RH, is the ratio of the amount of water vapor present in the air to the maximum amount of water vapor needed for saturation at a certain pressure and temperature. It is typically multiplied by 100 and expressed as a percent. Relative humidity shows how close the air is to being saturated, not how much water vapor the air contains. For this reason, RH is not a good indicator of the quantitative amount of water vapor in the air. It is only a relative measure that is highly dependent on the air temperature. Relative humidity greater than 100% is called supersaturation.
![]()
or
![]()
Dew Point Temperature
The dew point temperature, Td, is the temperature to which the air must be cooled to reach saturation, without changing the moisture or air pressure. It measures the actual moisture content of a parcel of air. Saturation occurs when the dew point temperature equals the air temperature.
![]()
![]()
When the dew point temperature is lower than the freezing point of water, it is also called the frost point.
Wet-Bulb Temperature
Wet-bulb temperature, Tw, is the lowest temperature that can be achieved if water evaporates within the air. When the relative humidity is 100%, the wet-bulb temperature is equal to the air temperature because there is no evaporation.
The wet-bulb temperature is difficult to calculate but easy to measure. To measure the wet-bulb temperature, all you need is a thermometer with a wet cloth wrapped around the bulb. Typically this thermometer is attached to an apparatus called a sling psychrometer to make it easy to spin around in the air to create lots of airflow over the wet cloth on the thermometer. The evaporation from the wet cloth cools the temperature measured, hence the wet-bulb temperature is always lower than the air temperature (or dry-bulb temperature) when relative humidity is less than 100%.
You can also estimate the wet bulb temperature using lines on a graph. Normand’s Rule is used to calculate the wet-bulb temperature from the air temperature and the dew point temperature. The wet-bulb temperature is always between the dew point and the dry-bulb temperature (Td ≤ Tw ≤ T). This can be implemented on thermodynamic diagrams, such as the Skew-T log P, which is discussed in more detail in the next chapter.
Take note of this description for later. To find the wet-bulb temperature on a Skew-T log P diagram, follow the dry adiabatic lapse rate line upward from the air temperature. Next, use the dew point temperature and follow an isohume (line of constant relative humidity) upward. The point where these two lines meet is called the lifting condensation level (LCL). From the meeting point, follow the moist (saturated) adiabatic lapse rate back down to obtain the wet-bulb temperature value. This is probably confusing at this point because we have not discussed the LCL or the moist adiabatic lapse rate, but don’t worry, we’ll repeat this logic again in the next chapter to make sure this is clear.
Why Do We Care So Much About Moisture?
You may be wondering at this point why we care so much about moisture and why we need so many definitions of (almost) the same thing. The reason is that moisture is an extremely important atmospheric property. Water can exist in three phases (vapor, liquid, ice) within the atmosphere at typical pressures and temperatures. It has an especially large impact on the human experience—think about a humid day, foggy conditions, rain, snow, or even hail! Less obvious is its impact on atmospheric stability, which drives the aforementioned conditions.
For now, let’s think about the process of water vapor condensing to form liquid water. There is one final definition of humidity that will be helpful.
Lifting Condensation Level
The lifting condensation level, zLCL, is the altitude where clouds form. At the LCL, temperature equals the dew point temperature, resulting in saturation and therefore condensation. The height (z) of the LCL is
![]()
where a is 0.125 km °C-1. We can also define the temperature at the LCL as follows.
![]()
Moist Adiabatic Lapse Rate
In the last chapter, we discussed how temperature changes as a dry parcel of air is lifted in the atmosphere. You will recall that as an air parcel is lifted, the temperature drops by 9.8 K every km due to the work the air parcel must do to the environment as it expands. Let’s add moisture to the discussion and see how this changes things.
If the air parcel reaches saturation (100% relative humidity) and water vapor condenses to liquid water within the parcel, latent heat will be released. In the case of a rising air parcel that is cooling from adiabatic expansion, this added heat from condensation counterbalances some of the cooling. Hence, the air parcel will no longer cool at the dry adiabatic lapse rate but at the smaller moist adiabatic lapse rate (Γm). Unlike the dry adiabatic lapse, the moist adiabatic lapse rate is not constant and varies based on the temperature and moisture of the air parcel.
We will approximate the moist adiabatic lapse rate with the following value.
![]()
The difference between the dry adiabatic lapse rate (Γm) and the moist adiabatic lapse rate (Γm) is significant and has profound influence on atmospheric stability, the topic of the following chapter.
Chapter 4: Questions to Consider
- Explain the conditions needed for saturation to occur.
- What is the saturation vapor pressure of air at 26°C?
- Explain the difference between specific humidity and relative humidity.
- If the temperature is 10°C and the pressure is 700 hPa, calculate the saturation specific humidity and the saturation mixing ratio.
- Explain why the moist adiabatic lapse rate is less than the dry adiabatic lapse rate.
Selected Practice Question Answers: