Terminating or Repeating?

Michelle Manes

Place Value and Decimals

Terminating or Repeating?

You’ve seen that when you write a fraction as a decimal, sometimes the decimal terminates, like:

$\frac 1 2 = 0.5 \quad \text{ and } \quad \frac {33} {1000} = 0.033.$

However, some fractions have decimal representations that go on forever in a repeating pattern, like:

$\frac 1 3 = 0.33333\dots \quad \text{ and } \quad \frac {6} {7} = 0.857142857142857142857142\dots.$

It’s not totally obvious, but it is true: Those are the only two things that can happen when you write a fraction as a decimal.

Of course, you can imagine (but never write down) a decimal that goes on forever but doesn’t repeat itself, for example:

$0.101001000100001000001\dots \quad \text{ and } \quad \pi = 3.14159265358979\dots.$

But these numbers can never be written as a nice fraction $\frac a b$ where $a$ and $b$ are whole numbers. They are called irrational numbers. The reason for this name: Fractions like $\frac a b$ are also called ratios. Irrational numbers cannot be expressed as a ratio of two whole numbers.

For now, we’ll think about the question: Which fractions have decimal representations that terminate, and which fractions have decimal representations that repeat forever? We’ll focus just on unit fractions.

Definition

A unit fraction is a fraction that has 1 in the numerator. It looks like $\frac 1 n$ for some whole number $n$ .

Think / Pair / Share

Which of the following fractions have infinitely long decimal representations and which do not?
$\frac 12 \qquad \frac 13 \qquad \frac 14 \qquad \frac15 \qquad \frac16 \qquad \frac 17 \qquad \frac 18 \qquad \frac 19 \qquad \frac 1{10}.$

Try some more examples on your own. Do you have a conjecture?

A fraction $\frac 1 b$ has an infinitely long decimal expansion if:

________________________________.

Problem 7

Complete the table below which shows the decimal expansion of unit fractions where the denominator is a power of 2. (You may want to use a calculator to compute the decimal representations. The point is to look for and then explain a pattern, rather than to compute by hand.)

Try even more examples until you can make a conjecture: What is the decimal representation of the unit fraction $\frac 1{2^n}$ ?

Fraction	Denominator	Decimal
$\frac 1 2$	$2$	$0.5$
$\frac 1 4$	$2^2$	$0.25$
$\frac 1 8$	$2^3$	$0.125$
$\frac 1 {16}$
$\frac 1 {32}$
$\frac 1 {64}$
$\frac 1 {128}$
$\frac 1 {256}$

Problem 8

Complete the table below which shows the decimal expansion of unit fractions where the denominator is a power of 5. (You may want to use a calculator to compute the decimal representations. The point is to look for and then explain a pattern, rather than to compute by hand.)

Try even more examples until you can make a conjecture: What is the decimal representation of the unit fraction $\frac 1{5^n}$ ?

Fraction	Denominator	Decimal
$\frac 1 5$	$5$	$0.2$
$\frac 1 {25}$	$5^2$	$0.04$
$\frac 1 {125}$	$5^3$
$\frac 1 {625}$
$\frac 1 {3125}$
$\frac 1 {15625}$

Marcus noticed a pattern in the table from Problem 7, but was having trouble explaining exactly what he noticed. Here’s what he said to his group:

I remembered that when we wrote fractions as decimals before, we tried to make the denominator into a power of ten. So we can do this:

$\frac12 = \frac12 \cdot \frac55 = \frac{5}{10} = 0.5.$

$\frac14 = \frac14 \cdot \frac{25}{25} = \frac{25}{100} = 0.25.$

$\frac18 = \frac18 \cdot \frac{125}{125} = \frac{125}{1000} = 0.125.$

When we only have 2’s, we can always turn them into 10’s by adding enough 5’s.

Think / Pair / Share

Write out several more examples of what Marcus discovered.
If Marcus had the unit fraction $\frac 1 {2^n}$ , what would be his first step to turn it into a decimal? What would the decimal expansion look like and why?
Now think about unit fractions with powers of 5 in the denominator. If Marcus had the unit fraction 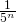$\frac 1{5^n}$ , what would be his first step to turn it into a decimal? What would the decimal expansion look like and why?

Marcus had a really good insight, but he didn’t explain it very well. He doesn’t really mean that we “turn 2’s into 10’s.” And he’s not doing any addition, so talking about “adding enough 5’s” is pretty confusing.

Problem 9

Complete the statement below by filling in the numerator of the fraction.

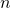The unit fraction $\frac 1{2^n}$ has a decimal representation that terminates. The representation will have $n$ decimal digits, and will be equivalent to the fraction

$\frac{?}{10^n}.$
Write a better version of Marcus’s explanation to justify why this fact is true.

Problem 10

Write a statement about the decimal representations of unit fractions $\frac 1{5^n}$ and justify that your statement is correct. (Use the statement in Problem 9 as a model.)

Problem 11

Each of the fractions listed below has a terminating decimal representation. Explain how you could know this for sure, without actually calculating the decimal representation.

$\frac 1{10} \qquad\quad \frac 1{20} \qquad\quad \frac 1{50} \qquad\quad \frac 1{200} \qquad\quad \frac 1{500} \qquad\quad \frac 1{4000}.$

The Period of a Repeating Decimal

If the denominator of a fraction can be factored into just 2’s and 5’s, you can always form an equivalent fraction where the denominator is a power of ten.

For example, if we start with the fraction

$\frac 1 {2^a 5^b},$

we can form an equivalent fraction

$\frac 1 {2^a 5^b} \ = \ \frac 1 {2^a 5^b} \cdot \frac {2^b 5^a} {2^b 5^a} \ = \ \frac {2^b 5^a} {2^{a+b} 5^{a+b}} \ = \ \frac {2^b 5^a} {10^{a+b}} .$

The denominator of this fraction is a power of ten, so the decimal expansion is finite with (at most) $a+b$ places.

What about fractions where the denominator has other prime factors besides 2’s and 5’s? Certainly we can’t turn the denominator into a power of 10, because powers of 10 have just 2’s and 5’s as their prime factors. So in this case the decimal expansion will go on forever. But why will it have a repeating pattern? And is there anything else interesting we can say in this case?

Definition

The period of a repeating decimal is the smallest number of digits that repeat.

For example, we saw that

$\frac 13 \ = \ 0.33333\dots = 0.\overline{3}.$

The repeating part is just the single digit 3, so the period of this repeating decimal is one.

Similarly, we know that

$\frac 67 \ =\ 0.857142857142857142857142\dots \ = \ 0.\overline{857142}.\phantom{857142857142857142}.$

The smallest repeating part is the digits $857142$ , so the period of this repeating decimal is 6.

You can think of it this way: the period is the length of the string of digits under the vinculum (the horizontal bar that indicates the repeating digits).

Problem 12

Complete the table below which shows the decimal expansion of unit fractions where the denominator has prime factors besides 2 and 5. (You may want to use a calculator to compute the decimal representations. The point is to look for and then explain a pattern, rather than to compute by hand.)

Try even more examples until you can make a conjecture: What can you say about the period of the fraction $\frac 1 n$ when $n$ has prime factors besides 2 and 5?

Fraction	Decimal	Period
$\frac 1 3$	$0.\overline{3}$	1
$\frac 1 6$	$0.1\overline{6}$	1
$\frac 1 7$	$0.\overline{142857}$	6
$\frac 1 {9}$
$\frac 1 {11}$
$\frac 1 {12}$
$\frac 1 {13}$
$\frac 1 {14}$

Imagine you are doing the “Dots & Boxes” division to compute the decimal representation of a unit fraction like $\frac 1 6$ . You start with a single dot in the ones box:

To find the decimal expansion, you “unexplode” dots, form groups of six, see how many dots are left, and repeat.

Draw your own pictures to follow along this explanation:

Picture 1: When you unexplode the first dot, you get 10 dots in the $\frac 1{10}$ box, which gives one group of six with remainder of 4.

Picture 2: When you unexplode those four dots, you get 40 dots in the $\frac 1{100}$ box, which gives six group of six with remainder of 4.

Picture 3: Unexplode those 4 dots to get 40 in the next box to the right.

Picture 4: Make six groups of 6 dots with remainder 4.

Since the remainder repeated (we got a remainder of 4 again), we can see that the process will now repeat forever:

unexplode 4 dots to get 40 in the next box to the right,
make six groups of 6 dots with remainder 4,
unexplode 4 dots to get 40 in the next box to the right,
make six groups of 6 dots with remainder 4,
and so on forever…

On Your Own

Work on the following exercises on your own or with a partner.

Use “Dots & Boxes” division to compute the decimal representation of $\frac 1{11}$ . Explain how you know for sure the process will repeat forever.
Use “Dots & Boxes” division to compute the decimal representation of 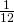$\frac 1{12}$ . Explain how you know for sure the process will repeat forever.
What are the possible remainders you can get when you use division to compute the fraction $\frac 1 7$ ? How can you be sure the process will eventually repeat?
What are the possible remainders you can get when you use division to compute the fraction $\frac 1 9$ ? How can you be sure the process will eventually repeat?

Problem 13

Suppose that $n$ is a whole number, and it has some prime factors besides 2’s and 5’s. Write a convincing argument that:

The decimal representation of $\frac 1 n$ will go on forever (it will not terminate).
The decimal representation of $\frac 1 n$ will be an infinite repeating decimal.
The period of the decimal representation of $\frac 1 n$ will be less than 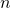$n$ .

Problem 14

Find the “decimal” expansion for 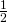$\frac 1 2$ in the following bases. Be sure to show your work:
$\text{two}, \quad \text{three}, \quad \text{four},\quad \text{five}, \quad \text{six}, \quad \text{seven}.$
Make a conjecture: If I write the decimal expansion of 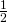$\frac 1 2$ in base 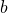$b$ , when will that expansion be finite and when will it be an infinite repeating decimal expansion?
Can you prove your conjecture is true?

License

Icon for the Creative Commons Attribution-ShareAlike 4.0 International License